File:Tetreal10bx10d.png
Original file (1,011 × 943 pixels, file size: 166 KB, MIME type: image/png)
Summary
| Title / Description
|
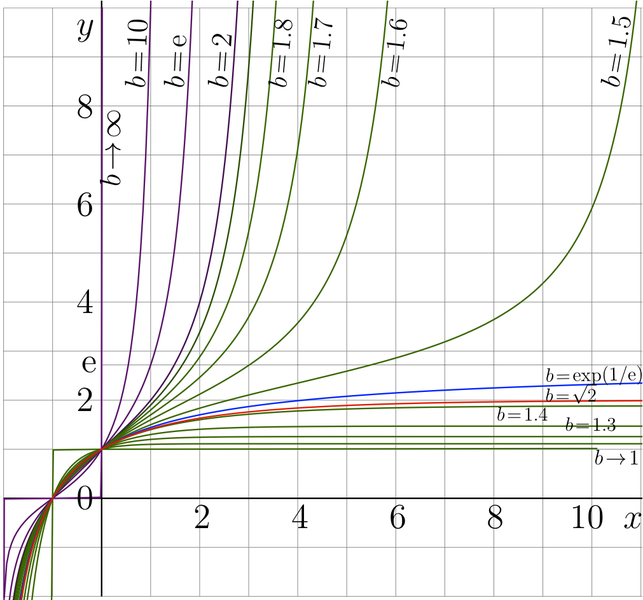
Real-real plot of tetration; y = tetb(x) versus x for various values of b > 1. |
|---|---|
| Citizendium author & Copyright holder
|
Copyright © Dmitrii Kouznetsov. See below for licence/re-use information. |
| Date created
|
2011 March |
| Country of first publication
|
Japan |
| Notes
|
Copy from http://tori.ils.uec.ac.jp/TORI/index.php/File:Tetreal10bx10d.png |
| Other versions
|
Similar up to date image is loaded at http://www.proofwiki.org/wiki/File:Tetreal10bx10d.png |
| Using this image on CZ
|
, then copy the code below to add this image to a Citizendium article, changing the size, alignment, and caption as necessary.
|
Please send email to manager A T citizendium.org .
C++ Generator
The lines at the figure can be generated with the C++ code below. For the compilation the following files should be loaded to the working directory: GLxw2048.inc, f2048ten.inc, f4ten.cin, fit1.cin
#include <math.h> #include <stdio.h> #include <stdlib.h> #define DB double #define DO(x,y) for(x=0;x<y;x++) using namespace std; #include <complex> typedef complex<double> z_type; #define Re(x) x.real() #define Im(x) x.imag() #define I z_type(0.,1.)
//b=10 #include "f4ten.cin"
#include "fit1.cin"
void ado(FILE *O, int X, int Y)
{ fprintf(O,"%c!PS-Adobe-2.0 EPSF-2.0\n",'%');
fprintf(O,"%c%cBoundingBox: 0 0 %d %d\n",'%','%',X,Y);
fprintf(O,"/M {moveto} bind def\n");
fprintf(O,"/L {lineto} bind def\n");
fprintf(O,"/S {stroke} bind def\n");
fprintf(O,"/s {show newpath} bind def\n");
fprintf(O,"/C {closepath} bind def\n");
fprintf(O,"/F {fill} bind def\n");
fprintf(O,"/o {.1 0 360 arc C S} bind def\n");
fprintf(O,"/times-Roman findfont 20 scalefont setfont\n");
fprintf(O,"/W {setlinewidth} bind def\n");
fprintf(O,"/RGB {setrgbcolor} bind def\n");}
//#include "ado.cin"
#define M(x,y) fprintf(o,"%6.4f %6.4f M\n",0.+x,0.+y); #define L(x,y) fprintf(o,"%6.4f %6.4f L\n",0.+x,0.+y); #define o(x,y) fprintf(o,"%6.4f %6.4f o\n",0.+x,0.+y);
main(){ int j,k,m,n; DB p,q,t1,t3,u,v,w,x,y; z_type z,c,d;
FILE *o;o=fopen("tet10bx.eps","w");ado(o,134,124);
fprintf(o,"22 22 translate\n 10 10 scale\n");
fprintf(o,"2 setlinecap\n");
for(m=-2;m<12;m++){if(m!=0){M(m,-2)L(m,10)}}
for(n= -2;n<11;n++){if(n!=0){M(-2,n)L(11,n)}} fprintf(o,".006 W 0 0 0 RGB S\n");
M(-2,0)L(11.1,0) M(0, -2)L(0,10.1) fprintf(o,".03 W 0 0 0 RGB S\n");
M(0,M_E)L(11.,M_E) fprintf(o,".006 W 0 0 0 RGB S\n");
fprintf(o,"1 setlinejoin 1 setlinecap\n");
//DO(m,400){x=-1.99+.008*m;y=Re(FIT1(log(10.),x)); if(y>10.3) break; if(m==0)M(x,y)else L(x,y)} fprintf(o,".03 W .5 0 .5 RGB S\n");
DO(m,400){x=-1.80+.02*m; y=Re(FIT1(log(2.),x)); if(y>10.3) break; if(m==0)M(x,y)else L(x,y)} fprintf(o,".03 W .5 0 .5 RGB S\n");
DO(m,400){x=-1.78+.02*m; y=Re(FIT1(log(1.9),x)); if(y>10.33) break; if(m==0)M(x,y)else L(x,y)} fprintf(o,".02 W 0 .5 0 RGB S\n");
DO(m,400){x=-1.77+.02*m; y=Re(FIT1(log(1.8),x)); if(y>10.33) break; if(m==0)M(x,y)else L(x,y)} fprintf(o,".02 W 0 .5 0 RGB S\n");
DO(m,400){x=-1.74+.02*m; y=Re(FIT1(log(1.7),x)); if(y>10.3) break; if(m==0)M(x,y)else L(x,y)} fprintf(o,".02 W 0 .5 0 RGB S\n");
DO(m,400){x=-1.72+.03*m; y=Re(FIT1(log(1.6),x)); if(y>10.3) break; if(m==0)M(x,y)else L(x,y)} fprintf(o,".02 W 0 .5 0 RGB S\n");
DO(m,400){x=-1.68+.04*m; y=Re(FIT1(log(1.5),x)); if(y>10.3) break; if(m==0)M(x,y)else L(x,y)} fprintf(o,".02 W 0 .5 0 RGB S\n");
DO(m,400){x=-1.64+.04*m; y=Re(FIT1(log(1.4),x)); if(x>11.1) break; if(m==0)M(x,y)else L(x,y)} fprintf(o,".02 W 0 .5 0 RGB S\n");
DO(m,400){x=-1.58+.04*m; y=Re(FIT1(log(1.3),x)); if(x>11.1) break; if(m==0)M(x,y)else L(x,y)} fprintf(o,".02 W 0 .5 0 RGB S\n");
DO(m,400){x=-1.52+.04*m; y=Re(FIT1(log(1.2),x)); if(x>11.1) break; if(m==0)M(x,y)else L(x,y)} fprintf(o,".02 W 0 .5 0 RGB S\n");
DO(m,400){x=-1.42+.04*m; y=Re(FIT1(log(1.1),x)); if(x>11.1) break; if(m==0)M(x,y)else L(x,y)} fprintf(o,".02 W 0 .5 0 RGB S\n");
//
DO(m,400){x=-1.65+.04*m; y=Re(FIT1(1./M_E,x)); if(x>11.1) break; if(m==0)M(x,y)else L(x,y)} fprintf(o,".03 W 0 0 .7 RGB S\n");
DO(m,400){x=-1.64+.04*m; y=Re(FIT1(log(sqrt(2.)),x)); if(x>11.1) break; if(m==0)M(x,y)else L(x,y)} fprintf(o,".03 W .8 0 0 RGB S\n");
DO(m,400){x=-1.873+.01*m; y=Re(FIT1(1.,x)); if(y>11) break; if(m==0)M(x,y)else L(x,y)} fprintf(o,".02 W 0 0 0 RGB S\n");
//
DO(m,401){x=-1.987+.01*m;y=Re(F4TEN(x));if(m==0)M(x,y)else L(x,y)}fprintf(o,".02 W .5 0 .5 RGB S\n");
//DO(m,39){x=-1.89+.098*m; y=Re(FSEXP(x));if(m==0)M(x,y)else L(x,y)} fprintf(o,".01 W 0 0 0 RGB S\n");
//DO(m,48){x=-1.82+.0973*m; y=Re(F2(x)); if(m==0)M(x,y)else L(x,y)} fprintf(o,".01 W 0 0 0 RGB S\n");
//DO(m,126){x=-1.72+.1*m; y=Re(F15(x)); if(m==0)M(x,y)else L(x,y)} fprintf(o,".01 W 0 0 0 RGB S\n");
//DO(m,130){x=-1.65+.1*m; y=Re(E1ETF(x)); if(m==0)M(x,y)else L(x,y)} fprintf(o,".01 W 0 0 0 RGB S\n");
//DO(m,130){x=-1.64+.1*m; y=Re(f21E(x)); if(m==0)M(x,y)else L(x,y)} fprintf(o,".01 W 0 0 0 RGB S\n");
M(-1.998,-2)L(-1.992,-.01)L(-1,0)L(-.01,.01)L(0,1)L(.012,10.1) fprintf(o,".02 W .4 0 .8 RGB S\n");
M(-1.01,-2)L(-1,0)L(-.99,.99)L(0,1)L(10.97,1.01) fprintf(o,".02 W .2 .4 0 RGB S\n");
fprintf(o,"showpage\n%cTrailer",'%'); fclose(o);
system("epstopdf tet10bx.eps");
system( "open tet10bx.pdf"); //mac
// system( "xpdf tet10bx.pdf"); // linux
getchar(); system("killall Preview");// mac
}
[[Latex] generator of labels
%If the curves are generated with the code above, the labels can be added with the Latex document below:
% \documentclass[12pt]{article} % <br> \usepackage{geometry} % See geometry.pdf % <br> \geometry{letterpaper} % ... or a4paper or a5paper or ... ??% <br> \usepackage{graphicx}% <br> \usepackage{amssymb}% <br> \usepackage{hyperref}% <br> \usepackage{rotating}% <br> \usepackage[utf8x]{inputenc}% <br> \usepackage[english,russian]{babel}% <br> \usepackage{color}% <br> \definecolor{red}{rgb}{1,0.1,0.1}% <br> \definecolor{black}{rgb}{0,0,0}% <br> \definecolor{white}{rgb}{1,1,1}% <br> \definecolor{yellow}{rgb}{1,.93,0}% <br> \definecolor{bluedark}{rgb}{0,0,.87}% <br> \paperwidth 528pt% <br> \paperheight 488pt% <br> \topmargin -102pt% <br> \oddsidemargin -78pt% <br> \textwidth 610pt% <br> \textheight 570pt% <br> % <br> \newcommand \sx {\scalebox}% <br> \newcommand \ing {\includegraphics}% <br> \newcommand \tet {\mathrm{tet}}% <br> \newcommand \pen {\mathrm{pen}}% <br> \newcommand \bC {\mathbb C}% <br> \newcommand \fac {\mathrm {Factorial}}% <br> \newcommand \rme {\mathrm e}% <br> \newcommand \rmi {\mathrm i}% <br> \newcommand \ds {\displaystyle}% <br> \newcommand \rot {\begin{rotate}}% <br> \newcommand \ero {\end{rotate}}% <br> % <br> \begin{document}% <br> \parindent 0pt% <br> \sx{4}% <br> {\normalsize% <br> \begin{picture}(155,122)% <br> \put(0,0){\ing{tet10bx}}% <br> %\put(-2,125){\sx{.7}{$y\!=\!{\rm tet}_{b}(x)$ ~as solution of~ $F(z\!+\!1)=\exp_b(F(z))$ ~,~ $F(0)\!=\!1$}}% <br> %\put( 0,125){\sx{.55}{$y\!=\!F(x)$ ~as solution of~ $F(z\!+\!1)\!=\!\exp_b(F(z))$ ,~ $F(0)\!=\!1$}}% <br> \put( 17,120){\sx{.6}{$y$}}% <br> \put( 17,100){\sx{.6}{$8$}}% <br> \put( 17,80){\sx{.6}{$6$}}% <br> \put( 17,60){\sx{.6}{$4$}}% <br> \put( 18,48){\sx{.6}{e}}% <br> \put( 17,40){\sx{.6}{$2$}}% <br> \put( 17,20){\sx{.6}{$0$}}% <br> \put( 20.4,16){\sx{.6}{$0$}}% <br> \put( 40.4,16){\sx{.6}{$2$}}% <br> \put( 60.4,16){\sx{.6}{$4$}}% <br> \put( 80.4,16){\sx{.6}{$6$}}% <br> \put(100.4,16){\sx{.6}{$8$}}% <br> \put(119,16){\sx{.6}{$10$}}% <br> \put(128.6,16.2){\sx{.6}{$x$}}% <br> %\put(2,90){\sx{.9}{$y\!=\!e\!\big(F_{1}(x\!+\!\rmi o)\big)$}}% <br> %\put(183,105){\sx{1.}{$y\!=\!F_{3}(x)$}}% <br> %\put(193,52){\sx{1.}{$y\!=\!\rme$}}% <br> \put(26.6,86){\sx{.5}{\rot{90} $b\!\rightarrow\!\infty$ \ero } }% <br> \put(31,106){\sx{.5}{\rot{88} $b\!=\!10$ \ero } }% <br> %\put(39.2,106){\sx{.5}{\rot{86} $b\!=\! 3$ \ero } }% <br> \put(42,87){\sx{.5}{\rot{87} $b\!=\! \rme$ \ero } }% <br> \put(48.4,106){\sx{.5}{\rot{86} $b\!=\!2$ \ero } }% <br> \put(53.3,106){\sx{.45}{\rot{85} $b\!=\!1.9$ \ero } }% <br> \put(60.3,106){\sx{.45}{\rot{85} $b\!=\!1.8$ \ero } }% <br> \put(68.4,106){\sx{.45}{\rot{84} $b\!=\!1.7$ \ero } }% <br> \put(83,106){\sx{.45}{\rot{83} $b\!=\!1.6$ \ero } }% <br> \put(128,106){\sx{.47}{\rot{80} $b\!=\!1.5$ \ero } }% <br> %\put(55,88){\sx{1.3}{$y\!=\!\tet_b(x)$ } }% <br> %\put(124,106){\sx{.6}{$b\!=\!1.5$}}% <br> \put(109,46){\sx{.4}{\rot{2}$b\!=\!\exp(1/\rme)$\ero}}% <br> \put(120,41.8){\sx{.34}{$b\!=\!\sqrt{2}$}}% <br> \put(120,38){\sx{.36}{$b\!=\!1.4$}}% <br> \put(120,33.6){\sx{.36}{$b\!=\!1.2$}}% <br> %\put(115,29.6){\sx{.36}{$b\!=\!1.1$}}% <br> \put(123,29){\sx{.36}{$b\!\rightarrow\!1$}}% <br> \end{picture}}% <br> \end{document}
%The generators are from TORI, http://mizugadro.mydns.jp/t/index.php/File:Tetreal10bx10d.png
Licensing
This media, Tetreal10bx10d.png, is licenced under the Creative Commons Attribution 3.0 Unported License
You are free:
To Share — To copy, distribute and transmit the work; To Remix — To adapt the work.
Under the following conditions:
Attribution — You must attribute the work in the manner specified by the author or licensor (but not in any way that suggests that they endorse you or your use of the work).
For any reuse or distribution, you must make clear to others the licence terms of this work (the best way to do this is with a link to this licence's web page). Any of the above conditions can be waived if you get permission from the copyright holder. Nothing in this licence impairs or restricts the author's moral rights.
Read the full licence.
References
https://www.morebooks.de/store/ru/book/Суперфункции/isbn/978-3-659-56202-0
http://www.ils.uec.ac.jp/~dima/BOOK/202.pdf
http://mizugadro.mydns.jp/BOOK/202.pdf Д.Кузнецов. Суперфункции. Lambert Academic Publishing, 2014, figure 17.1.
File history
Click on a date/time to view the file as it appeared at that time.
| Date/Time | Thumbnail | Dimensions | User | Comment | |
|---|---|---|---|---|---|
| current | 18:53, 11 March 2022 |  | 1,011 × 943 (166 KB) | Maintenance script (talk | contribs) | == Summary == Importing file |
You cannot overwrite this file.
File usage
The following 2 pages use this file:
