Energy (science)

Energy is a word with several connotations. The word goes back to the natural philosophy of Aristotle, ενέργεια (energeia), where it means roughly "efficacy". In the early nineteenth century the word was incorporated into science by Thomas Young. In science, the concept energy has a clear meaning, which, however, is not easy to explain because of the many forms in which energy manifests itself. The word energy is also very commonly used outside science, where it means physical or mental power to achieve something. "Negative energy" is a mental power that is in the way of achieving things.

In this article we will restrict attention to the scientific meaning of energy. In science, energy is a measurable property of a physical or chemical system, i.e., the energy of a system may be expressed by a single real number. Roughly speaking, the energy of a system is a measure of the amount of work that the system is able to perform on its environment. As stated, energy has many manifestations, be it chemical energy of a certain amount of gasoline, the kinetic energy of a moving cannon ball, the heat stored in a steam boiler, the potential energy of water in a reservoir, the fusion energy contained in a hydrogen bomb, the electricity in a battery. All these manifestations obey the same very important law: energy is conserved in conversion from one form of energy to the other. This law of conservation of energy is known as the first law of thermodynamics. This law pervades all of science, and is probably science's most important principle.

Let us consider an example. Assume we use a pump, running on gasoline, to pump water up to a reservoir, and when the reservoir is filled, we let the water flow down to drive an electrical generator. Doing this, we convert the chemical energy of the gasoline to (i) the mechanical energy of the pump to (ii) the potential energy of the water in the reservoir to (iii) the kinetic energy of the falling water, and finally to (iv) the electric energy generated by the generator. If we use the generated electric current for lighting, then the light bulbs convert the electric current to yet another form of energy, namely (v) light (electromagnetic radiation). During these energy conversion processes, the law of conservation of energy assures us that no energy is lost. To non-scientists the contrary may seem the case sometimes, because heat is generated (especially in burning the gasoline to drive the pump), and the heat will escape to the environment without any useful, or directly noticeable, effect. However, since heat is also a form of energy, it must be included in the energy balance of the first law.

Energy in classical mechanics

To keep the discussion simple we will consider a point particle of mass m in one-dimensional space. That is the position of m at time t is given by x(t). For more details and extension to the three-dimensional case, see classical mechanics. Let us assume that a force F(x) is acting on the particle. As an example we may think here of a mass in the gravitional field of the earth. The one dimensional space in this example is a line perpendicular to the surface of the earth. Actually, we will consider the slightly more complicated case of F being a function of x, because remember that the gravitational force F does not depend on x near the surface of the earth. (The expression for F close to the surface is: F = mg, where g is the gravitational acceleration, a quantity of approximate value 9.8 m/s².)

Potential energy

The physical system that we are considering is the particle of mass m in a force field F(x). Earlier we defined energy as the work that a system can deliver to its environment. If work is done by the system its energy decreases. If work is done on the system its energy increases. Think now, for example, of Galileo Galilei (GG), carrying mass up the stairs of the leaning tower of Pisa. Doing this, GG has the overcome the gravitational force, which works downward. The work ΔW performed by GG is proportional to the gain in height Δx and the absolute value |F| of the force. Since the work done by GG is positive and F is directed downward (F = − |F|), we have

$\Delta W=-F\Delta x\,,$

for the work done by GG on the system while carrying the mass up over a distance Δx. The gain in potential energy dU of the system is the work done on it by GG,

$dU=-Fdx\Longrightarrow U(x)=-\int _{x_{0}}^{x}\,F(x')\,dx',$

where we made the choice of zero of potential energy: $\scriptstyle U(x_{0})=0\,$. By the fundamental theorem of integral calculus, we have the important expression that relates force F(x) and potential energy U(x),

$F(x)=-{\frac {dU(x)}{dx}}.$

Kinetic energy

Besides potential energy, classical mechanics knows another form of energy: kinetic energy. Suppose GG drops the mass to the bottom of the tower after arriving at its top. The mass will pick up speed, (we will neglect air resistance, which will put some brake on the falling mass and generate some heat) and get the kinetic energy

$T\equiv {\tfrac {1}{2}}mv^{2}\quad {\hbox{with}}\quad v\equiv {\frac {dx}{dt}},$

where the speed of the particle is the absolute value of its velocity v.

Equivalence of kinetic and potential energy

This dropping of mass off the tower of Pisa is a good example of conversion of energy: potential energy is converted in kinetic energy. We will prove that energy is conserved, that is, the sum of kinetic and potential energy is constant in time.

${\frac {d}{dt}}(T+U)=mv{\frac {dv}{dt}}+{\frac {dU}{dx}}{\frac {dx}{dt}}=mva-Fv,\quad {\hbox{with}}\quad a\equiv {\frac {dv}{dt}},$

where a is the acceleration of the mass. Invoke Newton's second law (see classical mechanics):

$F=ma\,,$

and we have proven that the time derivative vanishes of the total energy E ≡ T + U of the physical system under consideration. That is, E is a conserved property of the system.

Collisions

Finally, one may wonder what happens when the particle, dropped by GG from the top of the tower of Pisa, hits the ground. Here we have a collision of two bodies, the earth and the dropped particle. The collision can be elastic, in which case no energy is dissipated. If we take the mass of the earth to be infinite, the particle bounces up with the same kinetic energy that it had when it hit the earth. That is, its speed |v | remains the same, but the sign of v changes. The momentum mv of the particle changes by −2mv on collision, which seems contradictory to the law of conservation of momentum. The latter conservation law holds when there are no outside forces acting on our physical system consisting of the earth and the dropped particle. Since we implicitly assumed that no outside forces are present, we indeed expect conservation of momentum. To explain this apparent violation, we note that the earth receives the absolute value of momentum M |V | = 2m|v | from the collision, where M is the mass of the earth and V is the velocity of the earth gained by the collision. When M goes to infinity, V goes to zero. Hence, for infinite mass the earth absorbs momentum without changing velocity and without picking up kinetic energy. This is why the kinetic energy of the bouncing particle is conserved.

A collision may be inelastic: the particle may break up in pieces which fly off with kinetic energy and the earth will absorb the remaining kinetic energy of the falling particle. This absorption is by increase of the internal energy of the earth, which in general implies some warming up of the earth. Of course, the law of energy conservation still holds: the kinetic energy of the broken particle pieces and the increase of the internal energy of the earth add up to the kinetic energy of the dropped particle.

As a final remark: most collisions are somewhere in between elastic and completely inelastic. The particle will bounce back some height, losing some kinetic energy that is transferred to the earth as an increase of the earth's internal energy. Also the internal energy of the dropped particle may increase somewhat by the collision. This must also be included in the energy balance.

Energy in thermodynamics

Heat

A thermodynamical system is a physical system with an extra property: temperature (T). When two thermodynamical systems of unequal temperature are in thermal contact, heat will flow spontaneously from the warmest (highest temperature) system to the coldest (lowest temperature) system. This heat flow will decrease the temperature of the warmer system and increase the temperature of the colder. The heat flow will be sustained until equilibrium is reached and the two systems have the same temperature. At equilibrium the spontaneous heat flow stops.

By using a heat pump it is possible to transfer energy from a colder to a warmer system. This requires input of mechanical or electrical work. The energy transferred from the colder to the warmer system is also called heat. Hence, heat is a form of energy transferred between two systems of unequal temperature.

Conversion of heat flow to work W.

Earlier we defined in a handwaving manner energy as the capacity of a system to do work. Now the question arises whether exchange of heat, which is an exchange of energy, can perform work. Or, in other words, can the energy content of a heat bath be utilized to perform work? It is clear that in any case two systems of different temperature are needed, otherwise heat will not flow. The first to recognize this clearly was William Thomson (Lord Kelvin).

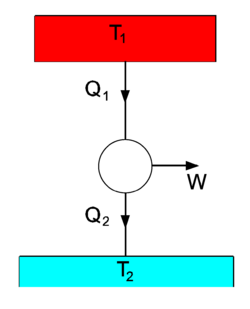
A spontaneous heat flow is depicted in the figure on the right, where we see two heat baths, with T₁ > T₂. The circle in the middle designates a heat engine, a cyclic process in which heat is converted into work W. From the first law of thermodynamics follows that after a number of full cycles of the engine, when no net energy is stored in the engine,

$Q_{1}-W-Q_{2}=0\Longrightarrow W=Q_{1}-Q_{2}.\qquad \qquad \qquad (1)$

The second law of thermodynamics states that

${\frac {Q_{2}}{T_{2}}}-{\frac {Q_{1}}{T_{1}}}\geq 0.\qquad \qquad \qquad \qquad \qquad \qquad \qquad \qquad \;(2)$

If we take the heat engine in the drawing to be the idealized Carnot engine that undergoes reversible changes, the equality sign holds. To obtain the theoretical upper bound to the efficiency of the process, we assume this to be the case. Multiplication of Eq. (2) by $\scriptstyle T_{2}/Q_{1}$ gives

${\frac {Q_{2}}{Q_{1}}}-{\frac {T_{2}}{T_{1}}}=0\Longrightarrow {\frac {Q_{2}-Q_{1}}{Q_{1}}}-{\frac {T_{2}-T_{1}}{T_{1}}}=0\Longrightarrow {\frac {W}{Q_{1}}}={\frac {T_{1}-T_{2}}{T_{1}}}.$

So, the work W is a fraction of the heat Q₁ delivered by the upper heat bath,

$W=\eta \,Q_{1}\quad {\hbox{with}}\quad \eta \equiv {\frac {T_{1}-T_{2}}{T_{1}}}\quad {\hbox{and}}\quad 0\leq \eta \leq 1.$

For instance, if T₁ = 500 ⁰C and T₂ = 20 ⁰C , then η = 480/(273.15+500) = 0.62. That is, at most 62% of the heat delivered by the upper heat bath is converted into work, the remaining energy is lost to the lower heat bath.

One may wonder why the work W is related here to Q₁. The answer is that the setup of the figure is a model for many engines. Historically, the model was first introduced for the steam engine. The upper heat bath is the steam boiler which is held at a constant temperature T₁ by burning fuel (in the days of the steam engine usually coal). During the cycle in the middle of the figure the steam drives a piston that performs work W. During this, the steam cools down, and the steam is cooled down even further in the condensor, becoming liquid water again. The condensor takes away the rest heat Q₂, which is not used any further, but given off to the environment (the lower heat bath of ambient temperature T₂). The condensed water is led back from the condensor to the steam boiler and heated again, completing the cycle. So, the heat flow between two reservoirs of unequal temperature (the steam boiler and the environment) generates work plus a rest energy Q₂. The fact that this rest energy appears has the consequence that only a fraction η of Q₁, the heat obtained from burning fuel, can be used to do work. Since the burning of fuel is the determining factor in the cost of operating the engine, the efficiency is expressed as a fraction of Q₁.

The same principles applies to combustion engines, for instance car engines, where the rest heat Q₂ is given off to the environment through the car radiator. The fact that only a fraction of the chemical energy stored in gasoline is converted into mechanical work (kinetic energy of the car) is not a design flaw, but a consequence of physical principles (the first and second law of thermodynamics).

The three arrows in the figure can be reverted, in which case the figure depicts a heat pump, for instance a refrigerator or an air conditioner. Work is delivered to the system, usually by an electric motor, and heat Q₂ is drawn from the lower temperature bath (for instance, the inside of a refrigerator). The heat Q₁ is transported to the higher temperature heat bath (in the case of a refrigerator the air in the kitchen, in the case of an air conditioner the outside air). Here we see an illustration of the Clausius principle: it takes work W to extract the amount Q₂ of heat from the low temperature bath. This is converted into the heat Q₁ that is transported to the high temperature bath. Since a refrigerator gives off its heat to the kitchen, it cannot be used as an air conditioner. The work W done by its electric motor is converted into the net heat Q₁ − Q₂. Overall, the refrigerator acts as an electric heater, converting electric energy W > 0 into the net heat Q₁ − Q₂ > 0 that is given off to the surroundings of the refrigerator. By the same reasoning it is clear why an air conditioner needs an outlet outside the house for its rest heat.

In practical applications, such as power plants and combustion engines, it is hard to achieve a large efficiency factor (T₁−T₂)/T₁, because T₂ is in practice always ambient temperature as it costs energy to obtain lower temperatures. The higher temperature T₁ cannot be raised too much as it is restricted by the burning process, the material of burners, etc. Power plants have a typical efficiency of 38%.

Work

Besides being able to exchange heat, a thermodynamic system can do also work on another system or on its environment, which decreases its internal energy U. Conversely, another system, or the environment, can do work on the system, increasing U. Above we already assumed that the exchange of energy by work was possible for the Carnot engine. Work can be mechanical, electrical, magnetic, chemical, and so on.

The standard textbook example of mechanical work regards a gas filled cylinder with a piston on top. Let the pressure inside the cylinder be p, the surface of the piston be S and the volume of the cylinder be V. If the piston is moved into cylinder over a distance Δx, an amount of work ΔW is performed on the gas which is equal to F Δx. By the definition of pressure the force F is equal to pS, so that the work is ΔW = pSΔx = pΔV, where we assume that p is constant under the small displacement of the piston. The internal energy increases by ΔU, while V decreases by ΔV, so that

$\Delta U=-p\Delta V\,.$

If the piston moves outward, the volume increases, the system perfoms work on its surroundings, costing it internal energy, and hence the sign in the equation covers this case as well.

The work performed on, or by, the system is of the form aΔb, where a does not depend on the size of the system (when we halve the volume of the system and its gas content the pressure p stays the same). The quantity a is an intensive parameter. The quantity b is linear in the size of the system, it is an extensive parameter. This is a general form for all expressions for work, they always involve an intensive/extensive parameter couple. Another example is the polarisation P (a macroscopic dipole) of a dielectric in a static electric field E. The work done by the field is EΔP. When we add an amount Δn mol of substance to a system, we increase its internal energy by μΔn, where μ is the chemical potential of the substance. This addition of substance can be seen as "chemical work" performed on the system. Even heat exchange fits this pattern, ΔQ = T ΔS, where the temperature T is an intensive and the entropy S is an extensive parameter.

Chemical energy

A chemical reaction

$\sum _{\mathrm {A} }n_{\mathrm {A} }\mathrm {A} \rightarrow \sum _{\mathrm {B} }n_{\mathrm {B} }\mathrm {B}$

may be exothermic, in which case heat escapes from the reaction in the form of translational (external) energy of the molecules B and often radiation. Or, the reaction may be endothermic in which case heat must be supplied in order to let the reaction proceed. Exothermic reactions form a source of chemical energy.

Very often chemical reactions proceed at constant—usually ambient—pressure p. The reaction heat Q is then equal to the change in enthalpy ΔH of the reactants. Indeed, according to the first law of thermodynamics, we have

$Q=U_{f}-U_{i}+p(V_{f}-V_{i})\equiv H_{f}-H_{i}.$

Here U _f is the total internal energy of the final product molecules B and U _i of the initial molecules A. Since the reaction occurs at constant pressure p, the work term is $\scriptstyle p(V_{f}-V_{i})$. This term must be included in the energy balance of the first law. The thermodynamic state function "enthalpy" is by definition H ≡ U + pV. Note that an exothermic reaction is characterized by H _f < H _i, i.e., has a negative reaction enthalpy Δ H ≡ H _f - H _i < 0. Correspondingly, an endothermic reaction has a positive reaction enthalpy.

In daily life the most important source of energy is the chemical energy obtained from the reaction called combustion. In this chemical reaction oxygen from the air reacts with a fuel, such as gasoline, coal, or natural gas, giving off heat. The fossil fuels contain carbon as the single most important element. Take graphite as an example:

C(graphite) + O₂(g) → CO₂(g)    ΔH = −393.6 kJ.

The second most important element contained in fossil fuels is hydrogen. The combustion reaction of gaseous hydrogen is

2H₂(g) + O₂(g) → 2H₂O(l)    ΔH = −571.6 kJ

Commercially available gasoline, diesel, LPG (liquified petroleum gas), etc. are mixtures of several hydrocarbons. Typical (approximate) values are

Combustion enthalpies MJ/kg

Natural Gas	48
Liquified petroleum gas	46
Aviation gasoline	44
Automotive gasoline	44
Kerosine	43
Diesel	42

These values are per kilogram. Ordinary gasoline has a density of 0.78 kg/L, so that 1 L of gasoline has an energy content of approximately 34 MJ/L.

It is of some interest to give a crude estimate of energy consumption in daily life, indicating orders of magnitude only. Assume, therefore, that it takes 0.1 L of gasoline to drive a midsized car one km (corresponds to 23 miles to the gallon, 10 km to the liter). This car consumes an energy of roughly 3.4 MJ per km, which is close to 1 KWh/km (kilowatt-hour per kilometer). Hence, driving the car over one kilometer costs roughly the same energy as running a 1000 W electric appliance for an hour. Of course, this does not take into account the energy loss in the generation of the electricity. A power plant running on fossil fuel has typically an efficiency of 38%. If we include this number, we see that driving a midsized car over one kilometer costs the same energy as running a 380 W electric apparatus for an hour.

Another example: Suppose the members of a houshold drive on average 30000 km (19000 mile) per year in a midsized car. This costs 30 MWh/year. The average Western-world household uses 3.5 MWh of electricity per year. Including the energy loss at the power station, a household spends three times more energy on driving a car than on electricity.

Electrostatic energy

Consider two point charges q₁ and q₂, a distance r₁₂ apart. By Coulomb's law the one particle acts on the other with a force that is inversely proportional to the mutual distance squared,

$F(r_{12})={\frac {q_{1}q_{2}}{4\pi \epsilon _{0}r_{12}^{2}}},$

where ε₀ is the vacuum permittivity. The forces on the two particles act along the line joining the particles. If the charges are of opposite charge, the forces are attractive, otherwise they are repulsive. As in classical mechanics, the work done by the force is minus force times distance. The work increases or decreases the potential energy of the system, so that the electrostatic energy of a system of two point charges is

$U(r_{12})=-\int _{\infty }^{r_{12}}{\frac {q_{1}q_{2}}{4\pi \epsilon _{0}(r'_{12})^{2}}}dr'_{12}={\frac {q_{1}q_{2}}{4\pi \epsilon _{0}r_{12}}}+U_{\infty }.$

The constant $\scriptstyle U_{\infty }$ can be chosen freely since its choice does not affect the electric field (minus the gradient of U), which is the physical quantity of concern. This freedom of choice is a form of gauge invariance. It is commmon to choose $\scriptstyle U_{\infty }=0$.

Consider next a system of N point charges. The potential energy of the system is additive, hence the electrostatic energy of a system of N point charges is,

$U=\sum _{i=1}^{N}\sum _{j>1}^{N}{\frac {q_{i}q_{j}}{4\pi \epsilon _{0}r_{ij}}}={\frac {1}{2}}\sum _{i=1}^{N}\sum _{j=1 \atop j\neq i}^{N}{\frac {q_{i}q_{j}}{4\pi \epsilon _{0}r_{ij}}}$

where the condition on the summation over j excludes the (infinite) self-energy. In the second equation the factor ½ is introduced to avoid counting the same interaction twice. This energy if of great importance in molecular physics, because a molecule can be seen as a collection of point charges.

This expression allows us to introduce a static potential (scalar) field due to a static charge distribution,

$V(\mathbf {r} )\equiv \sum _{i=1}^{N}{\frac {q_{i}}{4\pi \epsilon _{0}|\mathbf {r} _{i}-\mathbf {r} |}}.$

It is the work required to bring a single positive unit charge from infinity (where V is zero) to r. Or in other words, V(r) is the voltage difference between r and infinity, or, briefly, the electric potential at the point r due to the charge distribution.

Electric energy

Consider a conducting wire of finite length with a static voltage difference V between the ends. The voltage difference will be kept constant, for instance, by a battery, or an electric generator. An electric current (a flow of positive charges) will run from positive to negative voltage. This electric current transports energy,

$\Delta E=V\Delta Q\Longrightarrow {\frac {\Delta E}{\Delta t}}=V{\frac {\Delta Q}{\Delta t}}\Longrightarrow P=Vi.$

Here P is power (energy/time, expressed in watt), i is (direct) current (charge/time, expressed in ampere) and V is voltage difference (expressed in volt).

The magnitude i of the current is determined by the apparatus (light bulbs, electric oven, electric motors, etc.) that the wire runs through. All these will take up power. The power can be in the form of heat generated per unit time: i ²R, where R is the resistance of (part of) the wire. If, for instance, the current runs through an electric heater, part of the energy is converted to heat, i.e., electric power is converted into an energy flow from the heater outward, warming up the surroundings. If the current runs through an electric motor, electric power is converted to mechanical power, i.e., electrical energy is converted to mechanical work. In contrast to the conversion of heat to work, this process is practically loss-less. The only loss is by heating of the wires inside the motor, because of resistance of the wires (again i ²R).

In general, the energy carried by an electric current is measured in kWh (kilowatt×hour), instead of the regular SI unit of energy joule (J). Note that 1 kWh = 3600 kJ, since 1 W = 1 AV (ampere×volt) and 1 A = 1 coulomb/second (C/s) and 1 CV (coulomb×volt) = 1 J.

Equivalence of energy and mass

Einstein showed in his theory of special relativity that the energy of a free particle of (rest) mass m and speed v is equal to

$E=\gamma mc^{2}\quad {\hbox{with}}\quad \gamma ={\frac {1}{\sqrt {1-{\frac {v^{2}}{c^{2}}}}}},$

where c = 299 792 458 m/s is the speed of light in vacuum. Using the Taylor series

${\frac {1}{\sqrt {1+x}}}=1-{\frac {1}{2}}x+{\frac {3}{8}}x^{2}+\cdots ,$

we find that the energy of the free particle becomes

$E=mc^{2}+{\frac {1}{2}}mv^{2}+{\frac {3}{8}}mv^{4}/c^{2}+\cdots \,.$

Recalling that the energy of a free particle in Newton's classical mechanics is the kinetic energy ½mv², we see that Einstein discovered two completely new and unexpected facts. (i) The classical kinetic energy is the limit v << c, (if v << c neglect of third and higher terms in the expansion is allowed) and (ii) even a non-moving particle (v = 0) has energy. Especially this second fact has attracted much attention and its corresponding expression is the physics formula that is by far the best known among the general public.

Often Einstein's result is interpreted as mass depends on velocity, by defining the velocity dependent mass m(v) ≡ γm. This point of view shows that mass is not a conserved quantity, contradictory to what was postulated by the chemist John Dalton in the early nineteenth century. However, in contrast to mass, energy is conserved, provided we include relativistic energies E in a mass balance. If we have a system of particles with interaction U and total mass M then

${\frac {d}{dt}}(E+U)=0\Longrightarrow \Delta E=c^{2}\Delta M=-\Delta U.$

This equation is universal, in principle it is operative for chemical reactions, as well as for nuclear reactions. Let us first consider an example of the latter, the reaction of tritium (T) and deuterium (D) giving the isotope ⁴He and a neutron (n). This is the main reaction occurring in a hydrogen bomb explosion:

D + T → ⁴He + n + ΔU

Let us compute ΔU from a mass balance, where we use as unit of mass the unified atomic mass unit (u),

${\begin{matrix}{\hbox{Mass of}}&\mathrm {D} +\mathrm {T} :&2.014101778+3.0160492675&=5.0301510455\\{\hbox{Mass of}}&^{4}\mathrm {He} +\mathrm {n} :&4.002603250+1.0086641578&=5.0112674078\\\Delta M&&&\quad 0.0188836377\end{matrix}}$

The left hand side in the reaction equation has 0.01888 u more mass than the right hand side. To get an idea of the order of magnitude, we note that the mass of an electron m_e is 5.485 799 110× 10⁻⁴ u, so that ΔM is equal to 34.42 m_e, i.e., a little over the mass of 34 electrons. In the energy balance ΔM must appear as energy, noting that 1 u = 931.494013 MeV, we find that the energy that comes free in the reaction is ΔU = 17.59 MeV.

We may contrast this nuclear reaction to a typical chemical reaction,

H + H → H₂ + 4.5 eV

The left hand side (two free hydrogen atoms) has 4.5 eV more relativistic mass than the hydrogen molecule. The reaction energy 4.5 eV corresponds to 8.8× 10⁻⁶ m_e, which is a completely unobservable loss of mass. The fact that the mass of a molecule is less than the sum of masses of its constituting atoms is true, but such a small effect that it is never included, in, for instance, the translational or rotational energy of the molecule, where the molecular mass plays a role.

Energy in quantum mechanics

The energy of many (but not all) quantum mechanical systems is quantized, meaning that the energy of the system can take on only discrete values. The historic example of a quantum mechanical system with quantized energies is the one-dimensional harmonic oscillator. The energies are

$E_{n}=(n+{\tfrac {1}{2}})h\nu \quad {\hbox{with}}\quad n\in \mathbb {N} ,\quad {\hbox{i.e.,}}\quad n=0,1,2,\ldots ,$

where h is Planck's constant and ν is the fundamental frequency of the harmonic oscillator; h ν has the dimension energy. According to quantum mechanics it is impossible for the harmonic oscillator to have an energy equal to, e.g., 1.35 h ν, because there is no integer n such that n + ½ = 1.35. Max Planck was forced to introduce this quantized energy expression in his study of black body radiation, in which he assumed the walls of the black body to consist of thermally excited harmonic oscillators. This was the beginning of quantum theory.

As stated, not all energies are quantized, those of unbound systems (scattering systems) are not quantized. A well-known example is the ionization of the hydrogen atom, i.e., the removing of the electron of the H-atom. Once the electron has obtained an energy larger than the ionization potential (13.6 eV), it is a free electron—with a trajectory disturbed by the field of the H-nucleus—that can have any (non-quantized) energy.

In modern quantum mechanics, energy, as any observable physical quantity, is represented by a self-adjoint operator, usually designated by H in honor of William Rowan Hamilton. Most terms in the operator H are obtained from the corresponding classical Hamiltonian (the classical energy expressed in momenta and positions of the particles constituting the system). The momenta are replaced by gradients (times $\scriptstyle -i\hbar$ with $\scriptstyle \hbar =h/2\pi$) and the components of the position vectors are simply reinterpreted as multiplicative operators. Sometimes it is a matter of concern that operators do not commute, while the corresponding classical quantities always commute. Often one can then fall back on the Beltrami form of the Laplace operator for the kinetic energy. Further there are quantum mechanical energy terms that do not have classical counterparts. Commonly these terms depend on electron or nuclear spin. Spin terms can either be derived ad hoc (as in the Wolfgang Pauli's theory of electron spin), or more rigorously by Paul Dirac's relativistic theory. The energies E_n of the system appear as eigenvalues of the eigenvalue equation

$H\psi _{n}=E_{n}\psi _{n},\,$

the time-independent Schrödinger equation. By using n to label the eigenstates ψ_n, we may suggest the eigenvalues to be discrete, i.e., that n is integral. However, this is not necessary, n may be a continuous label. In that case ψ_n is usually not normalizable and is referred to as a scattering state.

In quantum mechanical studies the eigenvalue problem of any observable may appear occasionally. However, the observable H (energy) plays a very special—and central—role. Namely, it appears in the fundamental equation of quantum mechanics, Schrödinger's time-dependent equation,

$H\Psi =i\hbar {\frac {d\Psi }{dt}},$

which describes the time evolution of the state function Ψ. This equation is the quantum mechanical counterpart of Newton's second law in classical mechanics and Maxwell's equations in electrodynamics.

Literature

• Introduction to thermodynamics: P.W. Atkins, Physical Chemistry, Oxford University Press, 7th ed., ISBN 0198792859
• Introduction classical mechanics and electricity: R.A. Serway en J.W. Jewett, Physics for Scientists and Engineers, with Modern Physics, 6th edition, Thomson, 2004
• Quantum mechanics: Thomas Engel, Quantum Chemistry and Spectroscopy, PEARSON Benjamin Cummings