Chromatography theory
Chromatography is the process of separating a mixture components in solution based on their individual chemical and physical properties, characterized by a mobile fluid phase, and a stationary solid phase. The most common method is to use a packed column which the fluid is pumped through. The packing is determined by the type of chromatography used and the design requirements of the overall process.
Types of chromatography
Liquid Chromatography (LC) - this style is very versatile and easy to perform, but is limited by the high pressure drops encountered with pumping liquid through a dense packing.
Gas Chromatography (GC) - this method is used mostly for volatile organic compounds that are able to vaporize into a gaseous phase.
High Performance LC (HPLC) - the distinguishing feature of HPLC vs. LC is the ability of the former to handle very high pressures, allowing a much greater flow rate and better resolution of elution peaks.
Plate/Thin Layer Chromatography (PC/TLC) - this is a small scale method usually performed in a laboratory setting; it is based on capillary action of solvent rising up a thin coating of silica with solute dispersing as they rise.
Packed Column Chromatography
Single Component Concepts
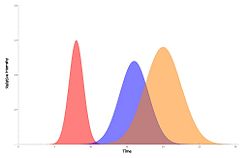
One of the most important characteristics of performing chromatography on a compound is its retention time. This is defined as the time it takes from the moment the compound is introduced to the column, to when the highest concentration is eluted out. It should be noted that it is not when the first measurable quantity is eluted.
If the column used is of sufficient length, the function of concentration vs. time should resemble a Gaussian distribution as the peak emerges, with retention time as its arithmetic mean. While the actual width of the peak can be ambiguous and hard to measure, the term “peak width” is defined as four standard deviations wide, and is used to estimate other quantities. The peak width can be calculated by finding the difference of time between the two points when the concentration was 0.607 (width at two standard deviations), and multiplying that quantity by two.
In the column, the packing used will occupy a certain volume called the void fraction, represented by ε. This is the space available for fluid flow, and so it has effects on both pressure drop and flow rate. As a packing ages, the void volume may increase due to compression from normal wear and tear.
Due to a large volume of the column being taken up by stationary packing, the change in fluid velocity must be accounted for.
- = retention time
- = total flow rate
- = "superficial velocity" =
- = void fraction =
- = interstitial velocity =
- = peak width =
As a component band moves through the column, it tends to spread out, so the times at which collection begins and ends can have a large effect on yield.
Multiple Component Concepts
When dealing with two or more distinct components of a mixture, the amount of separation of one from another is referred to as the resolution[1]. This is defined as:
- = Resolution =
A value of R=1 would mean that the theoretical beginning of the second curve would be at the exact same time as the theoretical ending of the first. However, since the elution curves have a much more Gaussian nature, there would still be an overlap of the two components.
Because of this, the purity (P) of the two is also used to evaluate the effectiveness of the separation.
- = purity of compound i = (mass of solute i collected)/(mass of all solutes)
Isotherms
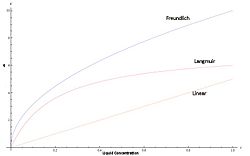
Isotherms represent the characteristic binding affinity of a solute in the mobile phase to the stationary packing. Depending on which isotherm models the target compound best, the elution peak and column dynamics may change.[2]
- Langmuir - Assumes a maximum possible loading that the solid phase can attain. Slope therefore goes to zero as mobile concentration increases.
- Freundlich - Not based on a physical model, but rather on empirical observations. Loading does not taper off, and goes to infinity at its limit. This is not physically possible, but it models typical concentrations very well.
- Linear - While not very common or valid for a wide variety of concentrations, most chromatography runs can use the linear approximation due to low concentrations typically used. This also yields the most accurate Gaussian peaks, and is the easiest to approximate
NTP and HTP Theory
While column chromatography is almost always performed with a continuous and unchanging column, two measures of a column’s performance are based on the theoretical premise of having multiple equilibrium stages of discreet height. These are referred to as the Number of Theoretical Plates (NTP) and the Height of Transfer Plates (HTP).
- = length of column
NTP is constant for a column for all components, regardless of what component you choose to calculate it with. HTP is also therefore a constant. NTP can be used to calculate resolution of two peaks if you only have the retention times.
Van Deemter Equation
To calculate the HTP based on the physical properties of the column and its packing, the Van Deemter Equation is used:
Where:
- is due to eddy diffusion in the packing
- is caused by longitudinal diffusion
- is governed by the mass transefer between mobile and stationary phases
- is the interstitial velocity of the mobile phase
- = particle shape
- = particle diameter
- = diffusion coefficient of mobile phase
- = diffusion coefficient of stationary phase
- = retention time of component
- = retention time of solvent