Lambert W function

The Lambert W function is used in mathematics to solve equations in which the unknown appears both as a linear term and in the argument of an exponential function or a logarithm, such as $3x+2=e^{x}$ or $x=\ln(4x)$. Such equations cannot otherwise, except in special cases, be solved explicitly in terms of algebraic operations, exponentials and logarithms.

Definition

The Lambert W function is defined as the multivalued function $W$ that satisfies

$z=W(z)e^{W(z)}\;$

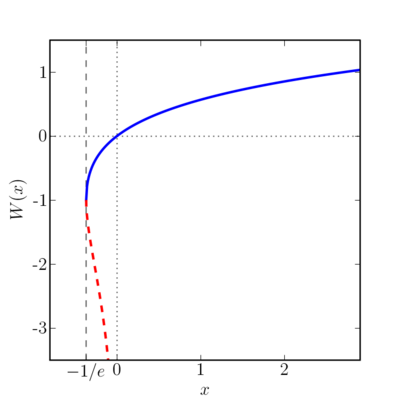
for any complex number $\scriptstyle z$. Equivalently, it may be defined as the inverse function of $f(w)=we^{w}$. An equation can be solved by rewriting it in the canonical form $C=we^{w}$, after which the solution is given by $\scriptstyle w=W(C)$. The multivaluedness of the Lambert W function means that the equations it may be used to solve may have more than one solution. The graph of the real-valued Lambert W function looks as follows:

The function has two real branches in the interval $-1/e<x<0$ which join at $x=-1/e$. Concretely, this means that the equation $x=we^{w}$ has two real solutions if $-1/e<x<0$. For example, if $x=-0.15$ (which is about half way between $-1/e$ and 0), there is one solution $\scriptstyle w_{0}\approx -0.17$ that lies on the blue graph and another solution $\scriptstyle w_{1}\approx -3.0$ that lies on the dashed red graph.

The single-valued function corresponding to the blue graph for Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \scriptstyle -1/e\, < \, x \,< \,\infty} is called the principal branch of the Lambert W function and is denoted by Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle W_0} . The single-valued function corresponding to the dashed red graph for Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle -1/e < x < 0} is called the negative branch. The negative branch asymptotically approaches Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \scriptstyle -\infty} as Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \scriptstyle x \to 0} while the principal branch grows unboundedly (although slowly) as Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \scriptstyle x \to \infty} .

History and application

Equations of the kind that can be solved analytically with the Lambert W function are common in mathematics and science, yet the utility of such a function was not realized until recently. The Lambert W function was introduced in the 1980's as a function in the Maple computer algebra system, whose interface required an explicit notation for solutions of equations. The function's history highlights the importance of good mathematical notation: due to previously not being recognized as a function in its own right, it had not been studied systematically, despite its most important properties requiring only elementary complex analysis. An account of the function and its history that helped popularize it is given in a 1996 paper by R. M. Corless et al. (with Donald Knuth a notable co-author).

The basic theory behind the Lambert W function was investigated in 1779 by Leonhard Euler. The Maple developers chose the name of Johann Heinrich Lambert instead of Euler's since Euler had referenced work by Lambert in his paper, and possibly because "naming yet another function after Euler would not be useful".

Since its introduction, the Lambert W function has been applied to problems ranging from quantum physics to the complexity of algorithms. Cranmer discusses the application of the Lambert W function in solar wind physics and writes in the conclusion: "The Lambert W function used in these solutions was defined and publicized only about a decade ago, but it has rapidly become a convenient tool for mathematical physicists. The elegance of explicit solutions to equations thought previously to be expressible only implicitly is clear, but there also are many practical benefits to having explicit solutions as well."

Mathematical details

To write:

• Taylor series
• Differential equation
• Integral
• Asymptotics
• Calculation

Particular values of the Lambert W function include Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle W(0) = 0} and Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle W(e) = 1} . The value

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle W(1) = 0.567143\ldots}

has been named the omega constant. Eric Weisstein notes that, as it satisfies Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle e^{-W(1)}=W(1)} , it "can be considered a sort of 'golden ratio' of exponentials".

See also

• Implicit function
• Elementary functions
• Catalog of special functions