Tetration: Difference between revisions

Revision as of 06:34, 29 October 2008

Tetration

\mathrm {tet} _{b}(x)

for

b=\mathrm {e}

,

b=2

,

b=\exp(1/\mathrm {e} )

, and

b={\sqrt {2}}

versus

x

.

This article is currently under construction. While, use article from wikipedia http://en.wikipedia.org/wiki/Tetration

Definiton

For real $b>1$ , Tetration $F=\mathrm {tet} _{b}$ on the base $b$ is function of complex variable, which is holomorphic at least in the range $\{z\in \mathbb {C} :~\Re (z)>-2\}$ , bounded in the range $\{z\in \mathbb {C} :~|\Re (z)|\leq 1\}$ , and satisfies conditions

F(z+1)=\exp _{b}\!{\big (}F(z){\big )}

F(0)=1

F\!{\big (}z^{*}{\big )}=F{\big (}z{\big )}^{*}

at least within range $\Re (z)>-2$ .

Real values of the arguments

Examples of behavior of this function at the real axis are shown in figure 1 for values $b=\mathrm {e}$ , $b=2$ , $b=\exp(1/\mathrm {e} )$ , and for $b={\sqrt {2}}$ . It has logarithmic singularity at $-2$ , and it is monotonously increasing function.

At $b\leq \exp(1/\mathrm {e} )$ tetration $\mathrm {tet} _{b}(x)$ approaches its limiting value as $x\rightarrow +\infty$ , and $\displaystyle \lim _{x\rightarrow +\infty }~\mathrm {tet} _{b}(x)>1$ .

At $b>\exp(1/\mathrm {e} )$ tetration $\mathrm {tet} _{b}(x)$ grows faster than any exponential function. For this reason the tetration is suggested for the representation of huge numbers in mathematics of computation. A number, that cannot be stored as floating point, could be stored as $\mathrm {tet} _{b}(x)$ for some standard value of $b$ (for example, $b=2$ or $b=\mathrm {e}$ ) and relatively moderate value of $x$ . The analytic properties of tetration could be used for the implementation of arithmetic operations without to convert numbers to the floating point representation.

Integer values of the argument

For integer $z$ , tetration

{\rm {tet}}_{b}

Etymology

Creation of word tetration is attributed to Englidh mathematician Reuben Louis Goodstein ^[1] ^[2].

Piecewice tetration

uxp

Analytic tetration

This section is not yet written. There is non-finished draft at User:Dmitrii Kouznetsov/Analytic Tetration.

Inverse of tetration

References

↑ "TETRATION, a term for repeated exponentiation, was introduced by Reuben Louis Goodstein". Earliest Known Uses of Some of the Words of Mathematics, http://members.aol.com/jeff570/t.html
↑ R.L.Goodstein (1947). "Transfinite ordinals in recursive number theory". Journal of Symbolic Logic 12.

Free online sources

http://reglos.de/lars/ffx.html , Lars Kindermann. References about Iterative Roots and Fractional Iteration.
http://math.eretrandre.org/tetrationforum/index.php , Discussion about tetration
http://tetration.itgo.com/ Tetration site by Andrew Robbins
http://www.tetration.org/ Tetration site by Daniel Geisler

[term-1] "TETRATION, a term for repeated exponentiation, was introduced by Reuben Louis Goodstein". Earliest Known Uses of Some of the Words of Mathematics, http://members.aol.com/jeff570/t.html

[good-2] R.L.Goodstein (1947). "Transfinite ordinals in recursive number theory". Journal of Symbolic Logic 12.

[1]

[2]

@@ Line 7: / Line 7: @@
 <math>x</math>.]]
 This article is currently [[under construction]]. While, use article from wikipedia http://en.wikipedia.org/wiki/Tetration
-==definiton==
+==Definiton==
+For real <math>b>1</math>, Tetration <math>F=\mathrm{tet}_b</math> on the base <math>b</math> is function of complex variable, which is [[holomorphic]] at least in the range
+<math> \{ z \in \mathbb{C} :~ \Re(z) > -2 \}</math>, bounded in the range
+<math> \{ z \in \mathbb{C} :~ |\Re(z)| \le 1 \}</math>, and satisfies conditions
+: <math>  F(z+1) = \exp_b\! \big( F(z) \big) </math>
+: <math>  F(0) = 1 </math>
+: <math>  F\!\big(z^*\big) = F\big(z\big)^*</math>
+at least within range <math> \Re(z)>-2 </math>.
+==Real values of the arguments==
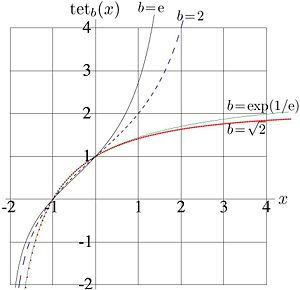
+Examples of behavior of this function at the real axis are shown in figure 1 for values
+<math>b=\mathrm{e}</math>,
+<math>b=2</math>,
+<math>b=\exp(1/\mathrm{e})</math>, and for
+<math>b=\sqrt{2}</math>. It has logarithmic singularity at <math>-2</math>, and it is monotonously increasing function.
+At <math>b\le \exp(1/\mathrm{e})</math> tetration <math>\mathrm{tet}_b(x)</math> approaches its limiting value as
+<math>x\rightarrow +\infty</math>, and <math>\displaystyle  \lim_{x \rightarrow +\infty}  ~ \mathrm{tet}_b(x) > 1</math>.
+At <math>b > \exp(1/\mathrm{e})</math> tetration <math>\mathrm{tet}_b(x)</math> grows faster than any exponential function. For this reason the tetration is suggested for the representation of huge numbers in [[mathematics of computation]].
+A number, that cannot be stored as [[floating point]], could be stored as <math>\mathrm{tet}_b(x)</math> for some standard value of <math>b</math> (for example, <math>b=2</math> or <math>b=\mathrm{e}</math>) and relatively moderate value of <math>x</math>. The analytic properties of tetration could be used for the implementation of arithmetic operations without to convert numbers to the floating point representation.
+==Integer values of the argument==
+For integer  <math>z</math>, tetration
+: <math>{\rm tet}_b</math>
 ==Etymology==

Tetration: Difference between revisions

Revision as of 06:34, 29 October 2008

Contents

Definiton

Real values of the arguments

Integer values of the argument

Etymology

Piecewice tetration

Analytic tetration

Inverse of tetration

See also

References

Free online sources

Navigation menu

Tetration: Difference between revisions

Revision as of 06:34, 29 October 2008

Definiton

Real values of the arguments

Integer values of the argument

Etymology

Piecewice tetration

Analytic tetration

Inverse of tetration

See also

References

Free online sources

Navigation menu

Search