Quadratic equation: Difference between revisions
imported>Aleksander Stos (formula not= equation) |
imported>Michael Underwood No edit summary |
||
| Line 1: | Line 1: | ||
{{subpages}} | {{subpages}} | ||
In [[mathematics]], or more specifically [[algebra]], | In [[mathematics]], or more specifically [[algebra]], a '''quadratic equation''' is one involving only [[polynomial]]s of the second degree. There exists a closed formula for finding the [[root_(mathematics)|root]]s of such an equation. It is therefore very useful for [[factoring]]. | ||
The formula is guaranteed to work for all quadratic polynomials, but sometimes the roots will be [[complex number]]s even when every other part of the problem deals only with [[real number]]s. | The formula is guaranteed to work for all quadratic polynomials, but sometimes the roots will be [[complex number]]s even when every other part of the problem deals only with [[real number]]s. | ||
For those keen on jumping to the answer, it states that the solutions to the equation <math>ax^2+bx+c=0</math> are given by | For those keen on jumping to the answer, it states that the solutions to the equation <math>ax^2+bx+c=0</math> are given by | ||
| Line 7: | Line 7: | ||
==The problem== | ==The problem== | ||
Any second-degree polynomial in the variable <math>x</math> will be of the form | Any real second-degree polynomial in the variable <math>x</math> will be of the form | ||
:<math>ax^2+bx+c\,</math> | :<math>ax^2+bx+c\,</math> | ||
where <math>a</math>, <math>b</math>, and <math>c</math> are real constants and <math>a</math> is not zero (if it was, the polynomial | where <math>a</math>, <math>b</math>, and <math>c</math> are real constants and <math>a</math> is not zero (if it was, the polynomial | ||
| Line 17: | Line 17: | ||
equals zero. | equals zero. | ||
The problem that the quadratic | The problem that the quadratic formula solves is to find those roots. | ||
==The solution== | ==The solution== | ||
The [[Fundamental Theorem of Algebra]] tells us that we should expect there to be two roots for a second-degree polynomial, | The [[Fundamental Theorem of Algebra]] tells us that we should expect there to be two roots for a second-degree polynomial, | ||
although they might be equal in some cases, and | although they might be equal in some cases, and may not be real even when the coefficients and variable are. If we call the roots <math>x_+</math> and <math>x_-</math> then what we are saying is that we have the quadratic equation | ||
:<math>ax_\pm^2+bx_\pm+c=0\ .</math> | :<math>ax_\pm^2+bx_\pm+c=0\ .</math> | ||
This is where the quadratic | This is where the quadratic formula comes in. It tells us that the solutions <math>x_+</math> and <math>x_-</math> can always be found as | ||
:<math>x_\pm=\frac{-b\pm\sqrt{b^2-4ac}}{2a}\ .</math> | :<math>x_\pm=\frac{-b\pm\sqrt{b^2-4ac}}{2a}\ .</math> | ||
| Line 41: | Line 41: | ||
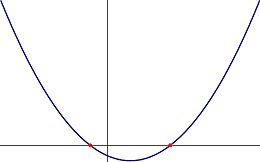
These can be seen graphically as the two red dots in Figure 1. | These can be seen graphically as the two red dots in Figure 1. | ||
In this case we can rewrite the polynomial in terms of its roots as | In this case we can rewrite the polynomial in terms of its roots as | ||
:<math>ax^2+bx+c = \left(x-x_+\right)\left(x-x_-\right)\ | :<math>ax^2+bx+c = \left(x-x_+\right)\left(x-x_-\right)\ ,</math> | ||
and it is easy to see that indeed if we set <math>x=x_+</math> or <math>x=x_-</math> then the polynomial well be equal to zero. | |||
===<math>\Delta=0</math>=== | ===<math>\Delta=0</math>=== | ||
| Line 48: | Line 49: | ||
and the polynomial can be expressed as | and the polynomial can be expressed as | ||
:<math>ax^2+bx+c = \left(x-x_0\right)^2\ .</math> | :<math>ax^2+bx+c = \left(x-x_0\right)^2\ .</math> | ||
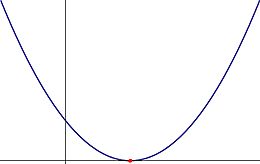
This case occurs when the parabola described by the polynomial just touches the <math>x</math>-axis at exactly one point, the red dot shown in Figure 2. | This case occurs when the parabola described by the polynomial just touches the <math>x</math>-axis at exactly one point, the red dot shown in Figure 2. Again, setting <math>x=x_0</math> in the polynomial makes it vanish. | ||
===<math>\Delta<0</math>=== | ===<math>\Delta<0</math>=== | ||
Revision as of 14:19, 13 October 2007
In mathematics, or more specifically algebra, a quadratic equation is one involving only polynomials of the second degree. There exists a closed formula for finding the roots of such an equation. It is therefore very useful for factoring. The formula is guaranteed to work for all quadratic polynomials, but sometimes the roots will be complex numbers even when every other part of the problem deals only with real numbers. For those keen on jumping to the answer, it states that the solutions to the equation Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle ax^2+bx+c=0} are given by
- Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle x=\frac{-b\pm\sqrt{b^2-4ac}}{2a}\ .}
For a more in-depth discussion of the problem, its solution, and their interpretations, read on.
The problem
Any real second-degree polynomial in the variable Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle x} will be of the form
- Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle ax^2+bx+c\,}
where Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle a} , Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle b} , and Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle c} are real constants and Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle a} is not zero (if it was, the polynomial would only be first-degree). In general the constant coefficients Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle a} , Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle b} , and Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle c} and the variable Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle x} can be complex, however the quadratic equation is most frequently applied and learnt in the case of real coefficients and a real variable so that is the case we will look at here. A polynomial of this form corresponds to a parabola, and the roots that the quadratic equation will give us are the values of Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle x} at which the parabola crosses the Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle x} -axis. This means that the roots of the polynomial are the particular values of Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle x} for which the polynomial equals zero.
The problem that the quadratic formula solves is to find those roots.
The solution
The Fundamental Theorem of Algebra tells us that we should expect there to be two roots for a second-degree polynomial, although they might be equal in some cases, and may not be real even when the coefficients and variable are. If we call the roots and Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle x_-} then what we are saying is that we have the quadratic equation
- Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle ax_\pm^2+bx_\pm+c=0\ .}
This is where the quadratic formula comes in. It tells us that the solutions Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle x_+} and Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle x_-} can always be found as
- Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle x_\pm=\frac{-b\pm\sqrt{b^2-4ac}}{2a}\ .}
Looking at the above result it is clear that the quantity Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \Delta= b^2-4ac} (called the discriminant) is of interest, for two reasons. First it is part of the quantity that is either positive or negative depending on whether you are looking at the root Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle x_+} or Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle x_-} , so it is this quantity that is responsible for whether we have two different roots or not. Second it is under a square root, so we must wonder what happens when it is negative. This gives us three cases to look at.
Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \Delta>0}
Here we have two distinct real roots, since the square root of Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \Delta} will also be real and greater than zero, meaning that we have
- Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle x_+=\frac{-b+\sqrt{b^2-4ac}}{2a}}
and
- Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle x_-=x_\pm=\frac{-b-\sqrt{b^2-4ac}}{2a}\ .}
These can be seen graphically as the two red dots in Figure 1. In this case we can rewrite the polynomial in terms of its roots as
- Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle ax^2+bx+c = \left(x-x_+\right)\left(x-x_-\right)\ ,}
and it is easy to see that indeed if we set Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle x=x_+} or Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle x=x_-} then the polynomial well be equal to zero.
Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \Delta=0}
Now there is only one distinct root. It is still real, and is said to have a multiplicity of 2. This is because the root is given by
- Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle x_0=\frac{-b}{2a}}
and the polynomial can be expressed as
- Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle ax^2+bx+c = \left(x-x_0\right)^2\ .}
This case occurs when the parabola described by the polynomial just touches the Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle x} -axis at exactly one point, the red dot shown in Figure 2. Again, setting Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle x=x_0} in the polynomial makes it vanish.
Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \Delta<0}
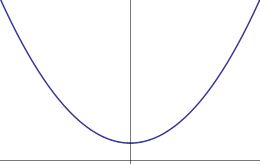
For negative values of the discriminant there are no real roots to the polynomial. Graphically this corresponds to the situation where the lowest point on the parabola is above the Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle x} -axis (or the highest point is below it, if Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle a} is negative) as shown in Figure 3. In this case the roots still exist, as guaranteed by the fundamental theorem of algebra, but they are complex so cannot be shown on the real number line.
Proof
The simplest way to show that the values Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle x_\pm} are in fact roots to the polynomial above is to substitute them into the equation
- Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \begin{align}ax_\pm^2+bx_\pm+c &=a\left(\frac{-b\pm\sqrt{b^2-4ac}}{2a}\right)^2+b\frac{-b\pm\sqrt{b^2-4ac}}{2a}+c \\ &=\frac{1}{4a}\left(b^2\pm2b\sqrt{b^2-4ac}+b^2-4ac\right)-\frac{b^2\pm b\sqrt{b^2-4ac}}{2a}+c \\ &=\frac{b^2\pm b\sqrt{b^2-4ac}}{2a}-c-\frac{b^2\pm b\sqrt{b^2-4ac}}{2a}+c \\ &=0\ , \end{align} }
as desired. Notice that this proof is valid even in the case where Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \Delta} is less than zero.