Group (mathematics)/Catalogs

The mathematical group concept represents a rather simple and natural generalization of common phenomena, so examples of groups are easily found, from all areas of mathematics.

Different classes of groups

Three different classes of groups are commonly studied:

• Finite discrete groups
• Infinite discrete groups
• Continuous groups

Examples of finite discrete groups

1. The trivial group consisting of just one element.
2. The group of order two, which f.i. can be represented by addition modulo 2 or the set {-1, 1} under multiplication.
3. The group of order three.
4. The cyclic group of order 4, which can be represented by addition modulo 4.
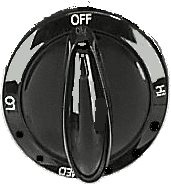
5. The noncyclic group of order 4, known as the "Klein viergruppe". A simple physical model of this group is two separate on-off switches.

Some physical models

Some common physical objects provide excellent introductions to group theory.

Model of the cyclic group of order 4. PD Image Example of group Z4. Let r1 be the act of turning the knob 1 step clockwise. Let r2 be the act of turning the knob 2 steps clockwise. Let r3 be the act of turning the knob 3 steps clockwise. Finally, let r0 be the act of just doing nothing. It's easy to see the following: Doing r1 and then r1 again gives the same result as doing r2. Doing r1 and then r2 gives the same result as doing r3. Doing r1 and then r3 gives the same result as doing nothing, i.e. r0. ... These results can be summarized in the following table: * r0 r1 r2 r3 r0 r0 r1 r2 r3 r1 r1 r2 r3 r0 r2 r2 r3 r0 r1 r3 r3 r0 r1 r2

Model of the non-cyclic group of order 4. PD Image Example of group Z2 x Z2. Let r01 be the act of flipping the right button. Let r10 be the act of flipping the left button. Let r11 be the act of flipping both buttons. Finally, let r00 be the act of just doing nothing. It's easy to see the following: Doing r01 and then r01 again gives the same result as doing r00, i.e. nothing. Doing r01 and then r10 gives the same result as doing r11. Doing r01 and then r11 gives the same result as doing r10. ... These results can be summarized in the following table: * r00 r01 r10 r11 r00 r00 r01 r10 r11 r01 r01 r00 r11 r10 r10 r10 r11 r00 r01 r11 r11 r10 r01 r00

Many examples of groups come from considering some object and a set of bijective functions from the object to itself, which preserve some structure that this object has.

• Topological groups:
  • Matrix groups; e.g the general linear group, the special linear group, the projective linear group and the Platonic groups
  • Abelian varieties.
    • Elliptic curves.
    • Abelian surfaces.
• Finite groups.
  • Cyclic groups.
  • Symmetric groups and alternating groups.
  • Dihedral groups.
  • Group of quarternions.
• Galois groups.
• Fundamental groups.