PH: Difference between revisions
imported>Milton Beychok m (Better to have a red link than sending reader to a disambiguation page to find the same red link) |
mNo edit summary |
||
| (8 intermediate revisions by 3 users not shown) | |||
| Line 2: | Line 2: | ||
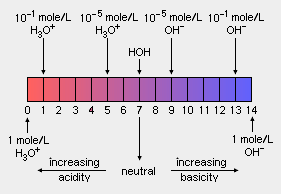
{{Image|PH scale.png|right|281px|The pH scale}} | {{Image|PH scale.png|right|281px|The pH scale}} | ||
The '''pH''' scale measures the [[acid|acidity]] or [[alkaline|alkalinity]] of an aqueous [[solution (chemistry)]], which is a solution in which water is the [[solvent]]. Values for pH range from about 0 (strongly acidic) to about 14 (strongly alkaline or [[base (chemistry)|basic]]). The pH of a neutral solution (neither acid or basic), such as pure [[water]] at room [[temperature]] and atmospheric [[pressure]] is 7, whereas the pH of an acidic solution is less than 7 and the pH of a basic solution is greater than 7. The pH scale is [[logarithm]]ic which means that a difference of one pH unit is equivalent to a ten-fold difference in hydrogen ion concentration. The notation '''pH''' is sometimes referred to as the '''''power of hydrogen''''' or the '''''potential of hydrogen'''''. | The '''pH''' scale measures the [[acid|acidity]] or [[alkaline|alkalinity]] of an aqueous [[solution (chemistry)|solution]], which is a solution in which water is the [[solvent]]. Values for pH range from about 0 (strongly acidic) to about 14 (strongly alkaline or [[base (chemistry)|basic]]). The pH of a neutral solution (neither acid or basic), such as pure [[water]] at room [[temperature]] and atmospheric [[pressure]] is 7, whereas the pH of an acidic solution is less than 7 and the pH of a basic solution is greater than 7. The pH scale is [[logarithm]]ic which means that a difference of one pH unit is equivalent to a ten-fold difference in hydrogen ion concentration. The notation '''pH''' is sometimes referred to as the '''''power of hydrogen''''' or the '''''potential of hydrogen'''''. | ||
The traditional way to determine whether a solution is acidic or basic is by wetting [[litmus paper]] with the solution. If the wet litmus paper turns red, the solution has a pH less than 7 and is acidic. If it turns blue, the solution has a pH greater than 7 and is acidic. Measuring the actual pH value of a solution is more accurately done with a [[pH meter]]. | The traditional way to determine whether a solution is acidic or basic is by wetting [[litmus paper]] with the solution. If the wet litmus paper turns red, the solution has a pH less than 7 and is acidic. If it turns blue, the solution has a pH greater than 7 and is acidic. Measuring the actual pH value of a solution is more accurately done with a [[pH meter]]. | ||
The pH scale was originally defined by [[Denmark|Danish]] biochemist [[Søren Peter Lauritz Sørensen]] in 1909, who wrote it as P<sub>H</sub>. It was subsequently changed to the modern notation of pH in 1920 by [[William Mansfield Clark]], an [[United States|American]] biochemist, for typographical convenience in his book ''The Determination of Hydrogen Ions''.<ref>{{cite book|author=William Mansfield Clark|title=The Determination of Hydrogen Ions|edition=1st Edition|publisher=William & Wilkins Company|year=1920|pages=page 35|id=}} Available in Google Books [http://books.google.com/books?id=spsLAQAAIAAJ&printsec=frontcover#v=onepage&q=&f=false here]</ref><ref>[http://science.jrank.org/pages/49372/pH.html pH: Potenz, The Determination of Hydrogen Ions, History of Analytical Chemistry, Electrochemistry, Past and Present] From the JRank Science & Philosophy website</ref> | The pH scale was originally defined by [[Denmark|Danish]] biochemist [[Søren Peter Lauritz Sørensen]] in 1909, who wrote it as P<sub>H</sub>. It was subsequently changed to the modern notation of pH in 1920 by [[William Mansfield Clark]], an [[United States of America|American]] biochemist, for typographical convenience in his book ''The Determination of Hydrogen Ions''.<ref>{{cite book|author=William Mansfield Clark|title=The Determination of Hydrogen Ions|edition=1st Edition|publisher=William & Wilkins Company|year=1920|pages=page 35|id=}} Available in Google Books [http://books.google.com/books?id=spsLAQAAIAAJ&printsec=frontcover#v=onepage&q=&f=false here]</ref><ref>[http://science.jrank.org/pages/49372/pH.html pH: Potenz, The Determination of Hydrogen Ions, History of Analytical Chemistry, Electrochemistry, Past and Present] From the JRank Science & Philosophy website</ref> | ||
==Definitions and discussion== | ==Definitions and discussion== | ||
| Line 14: | Line 14: | ||
{|class=wikitable border=1 align=right | {|class=wikitable border=1 align=right | ||
|+ H<sup>+</sup> and OH<sup>-</sup> ions<br/>molar concentrations vs. pH <ref name=Finesse>[http://finesse.com/files/pdfs/app-tech-notes/Finesse.TrupH.MeasureDef.pdf pH Measurement Definitions: The pH Scale]</ref> | |+ H<sup>+</sup> and OH<sup>-</sup> ions<br/>molar concentrations vs. pH <ref name=Finesse>[http://finesse.com/files/pdfs/app-tech-notes/Finesse.TrupH.MeasureDef.pdf pH Measurement Definitions: The pH Scale]</ref> | ||
!H<sup>+</sup> concentration<br/>(mole/litre!!OH<sup>-</sup> concentration<br/>(mole/litre)!!pH | !H<sup>+</sup> concentration<br/>(mole/litre)!!OH<sup>-</sup> concentration<br/>(mole/litre)!!pH | ||
|- | |- | ||
|1||0.00000000000001||align=center|0 | |1||0.00000000000001||align=center|0 | ||
| Line 50: | Line 50: | ||
A [[Molarity|molar]] [[Concentration (chemistry)|concentration]] of a compound, in [[Mole (unit)|moles]] per [[litre]] of solution, is commonly written as the symbol of the compound surrounded by square brackets. Thus, the [[hydrogen]] (H<sup>+</sup>) [[ion]] concentration in an aqueous solution is written simply as '''[H<sup>+</sup>]''' or as hydronium '''[H<sub>3</sub>O<sup>+</sup>]''' and both describe the equivalent entity.<ref>The hydronium notation reflects the physical situation, because the positive point charge H<sup>+</sup> binds strongly to H<sub>2</sub>O. Many textbooks therefore propagate the use of [H<sub>3</sub>O<sup>+</sup>].</ref> | A [[Molarity|molar]] [[Concentration (chemistry)|concentration]] of a compound, in [[Mole (unit)|moles]] per [[litre]] of solution, is commonly written as the symbol of the compound surrounded by square brackets. Thus, the [[hydrogen]] (H<sup>+</sup>) [[ion]] concentration in an aqueous solution is written simply as '''[H<sup>+</sup>]''' or as hydronium '''[H<sub>3</sub>O<sup>+</sup>]''' and both describe the equivalent entity.<ref>The hydronium notation reflects the physical situation, because the positive point charge H<sup>+</sup> binds strongly to H<sub>2</sub>O. Many textbooks therefore propagate the use of [H<sub>3</sub>O<sup>+</sup>].</ref> | ||
For very dilute solutions, the pH value can be defined by this simple expression:<ref name=Finesse/><ref>{{cite book|author=Darrell D. Ebbing and Mark S. Wrighton|title=General Chemistry|edition=2nd Edition|publisher=Houghton Mifflin|year=1987|pages=pp. 103-117|id=ISBN 0-395-35654-7}}</ref><ref>{{cite book|author=Kenneth W. Whitten and Kenneth D. Gailey|title=General Chemistry with Qualitative Analysis|edition=2nd Edition|publisher=Saunders College|year=1984|pages=pp. 263-278|id=ISBN 0-03-63287-5}}</ref><ref>[http://antoine.frostburg.edu/chem/senese/101/acidbase/faq/what-is-pH.shtml What is pH?] Professor Frederick A. Senese, [[Frostburg State University]], [[Maryland]]</ref> | For very dilute solutions, the pH value can be defined by this simple expression:<ref name=Finesse/><ref>{{cite book|author=Darrell D. Ebbing and Mark S. Wrighton|title=General Chemistry|edition=2nd Edition|publisher=Houghton Mifflin|year=1987|pages=pp. 103-117|id=ISBN 0-395-35654-7}}</ref><ref>{{cite book|author=Kenneth W. Whitten and Kenneth D. Gailey|title=General Chemistry with Qualitative Analysis|edition=2nd Edition|publisher=Saunders College|year=1984|pages=pp. 263-278|id=ISBN 0-03-63287-5}}</ref><ref>[http://antoine.frostburg.edu/chem/senese/101/acidbase/faq/what-is-pH.shtml What is pH?] Professor Frederick A. Senese, [[Frostburg State University]], [[Maryland (U.S. state)|Maryland]]</ref> | ||
:'''(1)''' <math>{\rm pH} = -\log_{10} \left[\rm H^+ \right] = \log_{10} \frac{1}{\left[\rm H^+ \right]}</math> | :'''(1)''' <math>{\rm pH} = -\log_{10} \left[\rm H^+ \right] = \log_{10} \frac{1}{\left[\rm H^+ \right]}</math> | ||
| Line 57: | Line 57: | ||
:'''(2)''' <math>{\rm pOH} = -\log_{10} \left[\rm OH^- \right] = \log_{10} \frac{1}{\left[\rm OH^- \right]}</math> | :'''(2)''' <math>{\rm pOH} = -\log_{10} \left[\rm OH^- \right] = \log_{10} \frac{1}{\left[\rm OH^- \right]}</math> | ||
Liquid water molecules undergo the following rapid, reversible dissociation (or ionization) reaction: | |||
:::2 H<sub>2</sub>O → H<sub>3</sub>O<sup>+</sup> + OH<sup>-</sup> | |||
This reaction can be called dissociation or self-ionization of water. Since both H<sub>3</sub>O<sup>+</sup> and OH<sup>-</sup> are simultaneously forming to a slight degree, this makes water both a very weak [[acid]] and a very weak [[Base (chemistry)|base]], with the same [[Acid dissociation constant|acid]] and [[base dissociation constant]], symbolized as K<sub>w</sub>. In the expression of this [[equilibrium constant]] for an aqueous solution, which can be called the dissociation or ionization constant of water, the [[molarity]] of water is omitted by convention. At about 25°C, K<sub>w</sub> = 1 x 10<sup><small>-14</small></sup>. The expression for K<sub>w</sub> is: | |||
:::K<sub>w</sub> = [H<sub>3</sub>O<sup>+</sup>] [OH<sup>-</sup>] = [H<sup>+</sup>] [OH<sup>-</sup>] = 1 x 10<sup><small>-14</small></sup> | |||
Since the product of the concentration of hydrogen ions and the concentration of hydroxide ions is a constant at about 25°C, namely: | Since the product of the concentration of hydrogen ions and the concentration of hydroxide ions is a constant at about 25°C, namely: | ||
| Line 64: | Line 73: | ||
taking logarithms gives: | taking logarithms gives: | ||
:'''(4)''' <math> | :'''(4)''' <math> \rm pH + \rm pOH = 14</math> | ||
At about 25°C, the mid-point of 7 in the pH scale indicates ionic neutrality of the solution, namely when [H<sup>+</sup>] equals [OH<sup>−</sup>] (see the adjacent table). An aqueous solution is ''[[acidic]]'' when [H<sup>+</sup>] > [OH<sup>-</sup>] and is ''[[Base (chemistry)|basic]]'' or ''alkaline'' when [OH<sup>-</sup>] > [H<sup>+</sup>]. | |||
As the theory behind [[chemical reaction]]s became more sophisticated, the definition of pH was reexamined. Specifically, as the role of electrical charge in chemical reactions became better understood, the definition of pH was changed to refer to the active hydrogen ion concentration. The more theoretical definition of pH, while not generally covered in many introductory chemistry textbooks, is the definition adopted by the [[International Union of Pure and Applied Chemistry]] (IUPAC):<ref name=Finesse/><ref name=IUPAC>[http://goldbook.iupac.org/P04524.html IUPAC Gold Book: pH]</ref> | As the theory behind [[chemical reaction]]s became more sophisticated, the definition of pH was reexamined. Specifically, as the role of electrical charge in chemical reactions became better understood, the definition of pH was changed to refer to the active hydrogen ion concentration. The more theoretical definition of pH, while not generally covered in many introductory chemistry textbooks, is the definition adopted by the [[International Union of Pure and Applied Chemistry]] (IUPAC):<ref name=Finesse/><ref name=IUPAC>[http://goldbook.iupac.org/P04524.html IUPAC Gold Book: pH]</ref> | ||
| Line 177: | Line 186: | ||
==References== | ==References== | ||
{{reflist}} | {{reflist}}[[Category:Suggestion Bot Tag]] | ||
Latest revision as of 12:01, 30 September 2024
The pH scale measures the acidity or alkalinity of an aqueous solution, which is a solution in which water is the solvent. Values for pH range from about 0 (strongly acidic) to about 14 (strongly alkaline or basic). The pH of a neutral solution (neither acid or basic), such as pure water at room temperature and atmospheric pressure is 7, whereas the pH of an acidic solution is less than 7 and the pH of a basic solution is greater than 7. The pH scale is logarithmic which means that a difference of one pH unit is equivalent to a ten-fold difference in hydrogen ion concentration. The notation pH is sometimes referred to as the power of hydrogen or the potential of hydrogen.
The traditional way to determine whether a solution is acidic or basic is by wetting litmus paper with the solution. If the wet litmus paper turns red, the solution has a pH less than 7 and is acidic. If it turns blue, the solution has a pH greater than 7 and is acidic. Measuring the actual pH value of a solution is more accurately done with a pH meter.
The pH scale was originally defined by Danish biochemist Søren Peter Lauritz Sørensen in 1909, who wrote it as PH. It was subsequently changed to the modern notation of pH in 1920 by William Mansfield Clark, an American biochemist, for typographical convenience in his book The Determination of Hydrogen Ions.[1][2]
Definitions and discussion
|
A molar concentration of a compound, in moles per litre of solution, is commonly written as the symbol of the compound surrounded by square brackets. Thus, the hydrogen (H+) ion concentration in an aqueous solution is written simply as [H+] or as hydronium [H3O+] and both describe the equivalent entity.[4]
For very dilute solutions, the pH value can be defined by this simple expression:[3][5][6][7]
- (1)
and the corresponding expression for the hydroxide (OH-) ions can be expressed as:
- (2)
Liquid water molecules undergo the following rapid, reversible dissociation (or ionization) reaction:
- 2 H2O → H3O+ + OH-
This reaction can be called dissociation or self-ionization of water. Since both H3O+ and OH- are simultaneously forming to a slight degree, this makes water both a very weak acid and a very weak base, with the same acid and base dissociation constant, symbolized as Kw. In the expression of this equilibrium constant for an aqueous solution, which can be called the dissociation or ionization constant of water, the molarity of water is omitted by convention. At about 25°C, Kw = 1 x 10-14. The expression for Kw is:
- Kw = [H3O+] [OH-] = [H+] [OH-] = 1 x 10-14
Since the product of the concentration of hydrogen ions and the concentration of hydroxide ions is a constant at about 25°C, namely:
- (3)
taking logarithms gives:
- (4)
At about 25°C, the mid-point of 7 in the pH scale indicates ionic neutrality of the solution, namely when [H+] equals [OH−] (see the adjacent table). An aqueous solution is acidic when [H+] > [OH-] and is basic or alkaline when [OH-] > [H+].
As the theory behind chemical reactions became more sophisticated, the definition of pH was reexamined. Specifically, as the role of electrical charge in chemical reactions became better understood, the definition of pH was changed to refer to the active hydrogen ion concentration. The more theoretical definition of pH, while not generally covered in many introductory chemistry textbooks, is the definition adopted by the International Union of Pure and Applied Chemistry (IUPAC):[3][8]
- (5)
where is the hydrogen ion activity and the factor is the hydrogen ion activity coefficient.[3][8]
Only in dilute solutions (about 0.001 moles per litre or less) are all anion and cations so far apart that they are free to be at their maximum activity where:
- (6) and thus
At higher acid and base concentrations, the space between cations and anions decreases, so that they begin to obstruct each other and shield each others charge. Thus, the mobility of the any particular ion is impaired by interactions with other ions and their associated electrical fields. These local electric field interactions affect the extent to which the ions can participate in chemical reactions, and give an apparent concentration that is always smaller than the real concentration, that is, the dimensionless parameter γ becomes less than unity. In other words, the ion activity is "slowed down" and [H+] becomes greater than aH+. The coefficient γ (ion activity over concentration) decreases with the increasing acid concentration. Therefore, for acid concentrations greater than about 0.001 moles per liter, it is advisable to use activities instead of concentrations in order to accurately predict pH.[3]
pH of some common substances
The two tables below list the pH ranges for each of a number of fairly common substances:
|
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||
| Notes: (1) Car battery acid is an aqueous solution of 65 weight % sulfuric acid, H2SO4 (2) Baking soda solution is an aqueous solution of sodium bicarbonate, NaHC03 (3) Milk of Magnesia is an aqueous solution of magnesium hydroxide, Mg(OH)2 (4) Household ammonia is ammonium hydroxide, NH4OH, a dilute aqueous solution of ammonia (5) Household lye is an aqueous solution of sodium hydroxide, NaOH | |||||||||||||||||||||||||||||||||||||||||||||||||||||||
References
- ↑ William Mansfield Clark (1920). The Determination of Hydrogen Ions, 1st Edition. William & Wilkins Company, page 35. Available in Google Books here
- ↑ pH: Potenz, The Determination of Hydrogen Ions, History of Analytical Chemistry, Electrochemistry, Past and Present From the JRank Science & Philosophy website
- ↑ Jump up to: 3.0 3.1 3.2 3.3 3.4 pH Measurement Definitions: The pH Scale
- ↑ The hydronium notation reflects the physical situation, because the positive point charge H+ binds strongly to H2O. Many textbooks therefore propagate the use of [H3O+].
- ↑ Darrell D. Ebbing and Mark S. Wrighton (1987). General Chemistry, 2nd Edition. Houghton Mifflin, pp. 103-117. ISBN 0-395-35654-7.
- ↑ Kenneth W. Whitten and Kenneth D. Gailey (1984). General Chemistry with Qualitative Analysis, 2nd Edition. Saunders College, pp. 263-278. ISBN 0-03-63287-5.
- ↑ What is pH? Professor Frederick A. Senese, Frostburg State University, Maryland
- ↑ Jump up to: 8.0 8.1 IUPAC Gold Book: pH
![{\displaystyle {\rm {pH}}=-\log _{10}\left[{\rm {H^{+}}}\right]=\log _{10}{\frac {1}{\left[{\rm {H^{+}}}\right]}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/976cd17230bab93ec437fce0bc287f9d5423381f)
![{\displaystyle {\rm {pOH}}=-\log _{10}\left[{\rm {OH^{-}}}\right]=\log _{10}{\frac {1}{\left[{\rm {OH^{-}}}\right]}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/6e6f4359383bd4196271be3665436854d4c7863f)
![{\displaystyle \left[{\rm {H^{+}}}\right]\left[{\rm {OH^{-}}}\right]=1\times 10^{-14}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/325d636951f2777f518ef1c863aa20753c214bea)

![{\displaystyle {\rm {pH}}=-\log _{10}\,(a_{\rm {H^{+}}})\equiv -\log _{10}\,\left(\gamma \,\left[{\rm {H^{+}}}\right]\right)}](https://wikimedia.org/api/rest_v1/media/math/render/svg/09ff183cee3b87fcc4935a330d77e07fcfab60ea)



![{\displaystyle a_{\rm {H^{+}}}=\left[{\rm {H^{+}}}\right]\,}](https://wikimedia.org/api/rest_v1/media/math/render/svg/4cd287a9a16a4326f0cf0eaeef60f51a297c6110)