Parallax: Difference between revisions
imported>Paul Wormer m (→Annual parallax: Added stellar parallax as synonym) |
imported>Caesar Schinas m (Bot: Update image code) |
||
| Line 1: | Line 1: | ||
{{subpages}} | {{subpages}} | ||
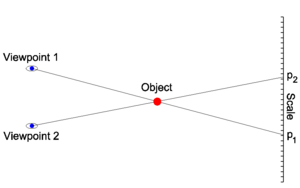
'''Parallax''' is the apparent change in the position of an object resulting from a change in position of the observer, see Fig. 1. | '''Parallax''' is the apparent change in the position of an object resulting from a change in position of the observer, see Fig. 1. | ||
{{Image|Parallax.png|right|300px|Fig. 1: Parallax. An observer at viewpoint 1 measures the object to be at p<sub>1</sub> on the scale and an observer at viewpoint 2 measures it at p<sub>2</sub>. The distance p<sub>2</sub>−p<sub>1</sub> is the (linear) parallax. }} | |||
==Diurnal parallax== | ==Diurnal parallax== | ||
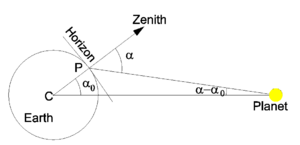
In [[astronomy]], the '''diurnal parallax''' is the parallax caused by the diurnal (daily) rotation of the Earth. In Fig. 2, an observer at ''P'' sees the surface of the Earth as a plane bounded by the horizon. Perpendicular to the plane is the [[zenith]]. The observer observes a planet (or another object in our solar system) under an angle α with the zenith, this angle is the ''topocentric zenith distance'' of the planet. The angle can be determined, for instance, against the background of fixed stars. Similarly, the angle α<sub>0</sub> is the ''geocentric zenith distance'' (measured from ''C'', the center of the Earth). The diurnal parallax is the angle α−α<sub>0</sub>. Note that the diurnal parallax is zero when the planet is in the zenith (above the observer at ''P''); both α and α<sub>0</sub> are zero. When the planet is at the horizon the diurnal parallax is maximum. When the planet is under the horizon the star cannot be observed at ''P''. Fig. 2 illustrates a theorem known from high-school geometry: any exterior angle of a triangle is equal to the sum of the two interior and opposite angles. | In [[astronomy]], the '''diurnal parallax''' is the parallax caused by the diurnal (daily) rotation of the Earth. In Fig. 2, an observer at ''P'' sees the surface of the Earth as a plane bounded by the horizon. Perpendicular to the plane is the [[zenith]]. The observer observes a planet (or another object in our solar system) under an angle α with the zenith, this angle is the ''topocentric zenith distance'' of the planet. The angle can be determined, for instance, against the background of fixed stars. Similarly, the angle α<sub>0</sub> is the ''geocentric zenith distance'' (measured from ''C'', the center of the Earth). The diurnal parallax is the angle α−α<sub>0</sub>. Note that the diurnal parallax is zero when the planet is in the zenith (above the observer at ''P''); both α and α<sub>0</sub> are zero. When the planet is at the horizon the diurnal parallax is maximum. When the planet is under the horizon the star cannot be observed at ''P''. Fig. 2 illustrates a theorem known from high-school geometry: any exterior angle of a triangle is equal to the sum of the two interior and opposite angles. | ||
{{Image|Diurnal parallax.png|left|300px|Fig. 2: Diurnal parallax is the angle α−α<sub>0</sub>.}} | |||
==Annual parallax== | ==Annual parallax== | ||
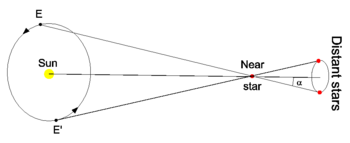
{{Image|Annual parallax.png|right|350px|Fig. 3: Annual stellar parallax is the angle α; E and E' are positions of the Earth half a year apart.}} | |||
Stars are too far removed to observe their diurnal parallax, the angles involved are too small. | Stars are too far removed to observe their diurnal parallax, the angles involved are too small. | ||
For near stars, however, it is possible to measure the '''annual parallax''' (also known as '''stellar parallax''') shown in Fig. 3. For an observer on Earth the near star seems to orbit during a year along a small ellipse when measured against the background of distant stars. The parallax of the distant stars is too small to be observed, so that they seem to be immobile. The ellipticity of the small ellipse depends on the angle between the plane of this ellipse and the plane of the Earth's orbit. The parallax angle α is used as a measure of distance: 1<nowiki>''</nowiki> (arc second) corresponds to a distance of 1 [[parsec]]. Note that a star nearer to the Sun has a larger parallax angle α, so that the Sun-star distance, measured in parsec, is inversely dependent on the angle α, measured in arc sec. | For near stars, however, it is possible to measure the '''annual parallax''' (also known as '''stellar parallax''') shown in Fig. 3. For an observer on Earth the near star seems to orbit during a year along a small ellipse when measured against the background of distant stars. The parallax of the distant stars is too small to be observed, so that they seem to be immobile. The ellipticity of the small ellipse depends on the angle between the plane of this ellipse and the plane of the Earth's orbit. The parallax angle α is used as a measure of distance: 1<nowiki>''</nowiki> (arc second) corresponds to a distance of 1 [[parsec]]. Note that a star nearer to the Sun has a larger parallax angle α, so that the Sun-star distance, measured in parsec, is inversely dependent on the angle α, measured in arc sec. | ||
Revision as of 11:45, 11 June 2009
Parallax is the apparent change in the position of an object resulting from a change in position of the observer, see Fig. 1.
Diurnal parallax
In astronomy, the diurnal parallax is the parallax caused by the diurnal (daily) rotation of the Earth. In Fig. 2, an observer at P sees the surface of the Earth as a plane bounded by the horizon. Perpendicular to the plane is the zenith. The observer observes a planet (or another object in our solar system) under an angle α with the zenith, this angle is the topocentric zenith distance of the planet. The angle can be determined, for instance, against the background of fixed stars. Similarly, the angle α0 is the geocentric zenith distance (measured from C, the center of the Earth). The diurnal parallax is the angle α−α0. Note that the diurnal parallax is zero when the planet is in the zenith (above the observer at P); both α and α0 are zero. When the planet is at the horizon the diurnal parallax is maximum. When the planet is under the horizon the star cannot be observed at P. Fig. 2 illustrates a theorem known from high-school geometry: any exterior angle of a triangle is equal to the sum of the two interior and opposite angles.
Annual parallax
Stars are too far removed to observe their diurnal parallax, the angles involved are too small. For near stars, however, it is possible to measure the annual parallax (also known as stellar parallax) shown in Fig. 3. For an observer on Earth the near star seems to orbit during a year along a small ellipse when measured against the background of distant stars. The parallax of the distant stars is too small to be observed, so that they seem to be immobile. The ellipticity of the small ellipse depends on the angle between the plane of this ellipse and the plane of the Earth's orbit. The parallax angle α is used as a measure of distance: 1'' (arc second) corresponds to a distance of 1 parsec. Note that a star nearer to the Sun has a larger parallax angle α, so that the Sun-star distance, measured in parsec, is inversely dependent on the angle α, measured in arc sec.